Experimentalphysik II
Betreuer/-innen für das AP gesucht

Allgemeine Kontaktinformationen:
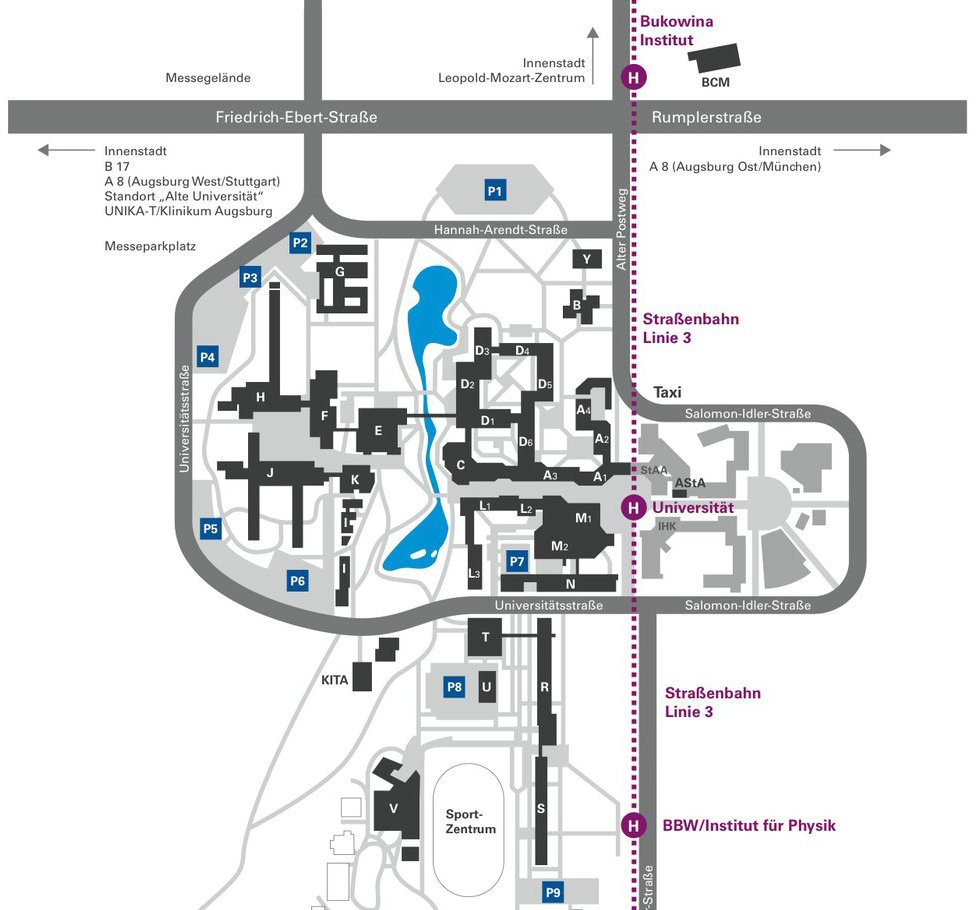
Anschrift:
Experimentalphysik II
Universität Augsburg
Universitätsstraße 1
86159 Augsburg
Deutschland
Telefon: +49 821 598 -3437 (Sekretariat)
Fax: +49 821 598 -3411
E-Mail: jutta.hampel@physik.uni-augsburg.de (Sekretariat)
Gebäude: R